- Interest Rate Supply And Demand
- Money Supply And Interest Rates Graph
- Money Supply And Real Interest Rates Chart
- Interest Rate And Money Demand
Interpretation
The real interest rate is calculated as the difference between the nominal interest rate and the inflation rate. The chart above displays the nominal interest rate of a 1-year US Treasury bond, the US inflation rate, and the resulting one-year real interest rate. Inflation is defined as the yearly percentage change of the Consumer Price Index (CPI). When inflation is high, prices for goods and services rise and thus the purchasing power per unit of currency decreases. The chart shows that, adjusted for inflation, the yields on US Treasuries (blue line) have often been negative.
Data Sources
- Nominal interest rate
- Robert Shiller Online Data: Nominal One-Year interest rate - from 1871 until 1929
- Capital Markets Data: Nominal One-Year interest rate - from 1929 until 1990
- Quandl: Constant Maturity Treasury Rate since 1990
- Inflation
- Robert Shiller Online Data: CPI from 1871 until 1913
- Federal Reserve Bank of St. Louis: CPI since 1913
Further Information
- Investopedia: What is a Real Interest Rate?
- Investopedia: What is Inflation?
- Longtermtrends: M2 Money Supply vs. Inflation
- Longtermtrends: US Treasury Yield Curve
- Longtermtrends: Bond Yield Spreads
The notion of a demand for money may strike you at first glance as bizarre. Don't you just want as much as you can get? Or isn't money what you use when you demand other goods? Here is where we have to remember that money is a stock
We can rearrange the equation to find real interest rate: Therefore, the real interest rate, or actual return on investment, of the portfolio equals: The real interest that Sam’s investment portfolio earned last year, after accounting for inflation, is 1.26%. Related Readings.
not a flow, and that income and wealth are not money. Demand for money is a question of how much of your wealth you wish to hold in the form of money at any point in time. (Supply of money is also a stock concept.)Your demand for money is how much of your wealth you wish to hold as money at any moment in time. It is thus a stock demand. Your wealth is a stock, and you must decide how to allocate that stock of wealth between different kinds of assets -- for example a house, income-earning securities, a checking account, and cash.
Why would you hold any of your wealth as money -- as cash or checking deposits? Those assets earn little or no interest. Wouldn't it be more sensible to hold all your wealth in the form of assets that yield income? Note that:
- 1. There is a cost associated with holding money balances (you give up interest payments),
2. There is no intrinsic value in the money balances you hold except in their use as a medium of exchange. Generally, you acquire money in order to get rid of it -- to buy things. While you hold it, money does not keep you warm, entertain you, or provide any other benefit.
3.2 Transactions Motive for Holding Money
The most obvious answer is that we hold some money because it's convenient to buy stuff with. We'll call this first reason the transactions motive. Essentially, it's convenient to hold a certain average amount of money at any given time, depending on the kind of purchases you make and the size of your income.
One of the most important functions of money is that it is the universally accepted medium of exchange -- this is the main reason you hold money. Thus, one reason to hold money is to use it as a means of payment in transactions in the future. Now, if there was a perfect match between the moments you receive money in transactions and the moments you use money, you would not need to hold any money at all. If I were paid every Friday, and I could pay all my bills on the same day, then I would need to hold very little money.
Unfortunately, in the real world, there is not going to be an exact match between when I receive money and when I need to make payments. Let us say that as a work study student, you receive $500 every month as payment for your work. This payment comes once a month. But you need to pay for rent, food, movies, books, copying, pens etc. This is spread out over the month. So, on the first of the month, you deposit $500 in you bank account at Fulton bank, and then you withdraw this money and run your account down to zero over the course of the month. In the course of the month, you hove an average money holding of $250. Same thing happens the next month, and the next, over the year.
Your average holding of money then, is $250: this is how much you have on average in your bank account over the course of a year.
If instead, you were paid $250 every two weeks, then you would hold $250 at the beginning of the two weeks, and run it down to zero over the course of two weeks, repeat this the next two weeks, and so on.
Can you see that on average, you would be holding $125 in your account?
In other words, the need you have for holding money balances will depend on the smoothness with which the time you get paid and the time you use the money to make payments mesh. Thus, the need to hold money balances is in part a result of the institutional payments mechanisms in the economy. An agrarian day laborer in India does not hold very much cash balances because she will get paid on a daily basis in small amounts and then use up the money she receives to pay for her transactions of purchasing food almost immediately. She will have a near zero holding of money balances. But in a system where people are paid with longer term contracts, regularized wages and salaries, and where they get paid in intervals of a week, a fortnight or a month, and where incomes are relatively stable, the need to hold money balances will be higher.
Even if there was not a perfect match between my receipts of cash and the moments in which I use it as a means of payments for transactions, if I could costlessly and immediately convert any bonds I hold into money, then there is no reason to hold money. I would hold all wealth as bonds, and sell a bond for money the moment I need to make a purchase, holding money for only an instant.
So for example, you are paid $500 per month. You hold an average balance of $250 as you start out the month with a $500 bank balance, and then run to down over the month. But what if you find that you can buy a $250 bond at the beginning of the month, and sell it in the middle of the month, earning an interest for half the month? When you get paid, you could put $250 in your checking account as a money holding, and buy a bond for $250. At the end of two weeks, when your $250 has been used up, you can sell the bond and deposit another $250 in your account. Your average money holdings have just dropped to $125, and you earn interest on $250 bonds every fortnight.
You can take this further an further. Why not deposit only $125, and buy $375 worth of bonds? Sell $125 of bonds every week, and earn interest for three weeks. In effect, the question is:
Why hold any money balances at all? Why not always hold bonds and only get hold of money the moment you need it to pay for transactions?
It is costly, in terms of time and resources, to keep moving in and out of bonds or other assets and money. Since this is the case, I will desire to hold a certain level of money balances on average, to meet my needs to pay for transactions. This is called the transactions demand for money.
If the interest payments I receive on bonds and other assets is high, then it is worth my while to move in and out of stocks and bonds and money, so that I can earn this interest payment instead of holding money balances. If the interest rate is not that high, then it is not worth it to move in and out of money and bonds in order to receive this interest payment.
Another way to look at it is that the interest rate describes the cost of holding money balances. This is because the interest rate tells you the amount of interest income you have to forego by holding money balances instead of lending out that money and holding an asset like a bond.
This is enough to generate a curve which plots the demand for money -- the amount you wish to hold as opposed to holding wealth as bonds -- as a function of r. This curve will slope downward.
Caution: This looks like the kind of demand curve you're used to in micro. It isn't. Those represent demand for a flow of a good. This shows what stock of money people wish to hold as part of their asset portfolios.
We emphasize the way that r affects transactions demand because it's important to our money market story. But two other things will also affect transactions demand. If income changes, transactions demand should change with it. As your income rises so do your expenditures, and hence the amount of wealth you might want to hold as money at any instant in time. Similarly it's reasonable to assume that at a national level, demand for money will grow as national income grows, and decline if national income declines. Additionally, as the overall price level of goods and services changes, transactions demand will change with it: if you hold money to buy stuff, and it becomes less expensive to buy stuff, you'll hold less money.
So the transactions demand for money depends on three things:
a) interest rate: as we have noted above, the interest rate is in effect the price of holding money balances. It is the income I forego when I hold money balances. If the interest rate goes up, then the returns on moving in and out of money into other assets and back will increase, so people will hold a lower level of money balances. If the interest rate falls, then the returns on moving out of money balances and into assets are not so great. In this case, it is not worth it to move out of money into other assets and then back when you need to make payments on transactions, so you will hold a higher level of money balances.
b) aggregate income: if the volume of income and output produced in the goods markets increases, then clearly there will be a larger volume of transactions and exchanges taking place. People will need to hold a larger volume of money to meet all these transactions and make payments.
c) price level: if prices rise, then people will need to hold a higher level of money balances to meet their payments transactions. If prices fall, people will need a lower volume of money balances to support a given level of transactions.
3.3 Speculative Motive for Holding Money
Now, in addition to the transactions motive, there is one other reason why people have a demand for holding money balances. This is called the speculative motive. Suppose that interest rates fluctuate. At a two percent rate of interest, you would get $1,020 in a year's time in exchange for $1,000 in cash now (i.e. by buying now for $1,000 a bond that pays $1,020 in a year, which is the same thing as lending $1,000 at two percent interest). Suppose that the rate of interest is now two percent, but you expect it to rise to ten percent shortly. At ten percent, $1,000 in cash now will get you $1,100 in a year's time. So if you think interest rates re unusually low and likely to rise, you might keep your wealth as money rather then buying bonds at the low current interest rate.
Another way to think about this, which will become clearer after you finish the next section, is that if you think that interest rates will rise, then any bonds you buy now at two percent interest will fall in value after the interest rate rises. 'Speculative' simply means speculating -- gambling, if you like -- that the value of an asset will change and you can profit by it. Usually we think of speculating in terms of buying an asset: if I expect that real estate in Carlisle is about to rise in value, I might buy some in hope of selling after the price rises. But if I think that an asset's price is about to fall, I can also speculate by holding cash, so that I can buy it after the price drops.
Of course if I think that interest rates are unusually high, then I will buy as many bonds as possible to lock in the higher interest rate. Another way to put it is that after the interest rate falls, these bonds will be worth more.
To recapitulate: in general, when interest rates are high, people speculate that they will not stay high, but will fall. If this is the case, then people will demand less money holdings and move into bonds. When (or if) interest rates do fall, their bonds will rise in value.
But, if interest rates are low, people expect that they will go up. So they prefer to hold on to money balances, and will move out of bonds, for fear that the value of those bonds will fall when (or if) interest rates rise in the future.
3.4 Money Demand as a Function of the Interest Rate
So far, we have two reasons why the amount of money that people wish to hold might vary with the interest rate. It happens that they both agree about the nature of the change: at low interest rates money demand will be high, at high interest rates the amount ot their portfolios that people wish to hold as money will be low.
Before we put this together with the supply of money, we need to go over the relation between the interest rate and the price of bonds.
3.5 Bond Prices and the Interest Rate
The final link in this story is that the fluctuations in assets prices are intimately linked to the interest rate. This is because the reason to purchase an asset like a bond and to agree to loan out your money is that your plan to earn an interest on the loan. Thus, the price of a bond is linked to the interest that it promises to give the lender.
There are several ways to think about this. One is to remember that a bond is nothing but a promise to make future payments -- a piece of paper that gives you the right to get certain payments of money at certain future times.
The price of a bond is simply the amount of money one can sell it for right now. This will affect both the 'primary' market in bonds -- firms selling bonds to raise money to buy capital goods, or government selling bonds to finance a fiscal deficit -- as well as the 'secondary' market -- people buying and selling previously-issued bonds. If I want to buy a one-year bond, then a new one-year bond issued by the U.S. government, and a 2-year bond issued a year ago whose holder wishes to sell it, are the same thing as fas as I'm concerned -- both are promises to pay money in a year's time.An 'interest rate' is just a different way of discussing the price. To get an interest rate, we subtract the money paid now for the bond from the money the bond promises to pay later, and call that difference 'interest.' If we express that 'interest' as a percentage of the money paid now for the bond, we have an interest rate. So if the money that the bond pays in the future is given, then the higher the price (in money now) of the bond, the lower the difference between that price and what the bond pays later, so the less the interest and hence interest rate.
Here is the same idea in more general mathematical language.Let us say that the interest rate is r%. If you lend $B today, then you will get:
A = B (1 + r)Mtwhere A is the amount you get back at the end of t years, B is the amount you loaned today, and r is the interest rate you receive.
Now, a bond is basically a promise to pay an amount A at the end of t years.
Let us say you bought a bond that promised to pay you $110 at the end of one year. If you bought that bond for $100, then in effect, you loaned out $100 and got back $110 at the end of one year. You got $10 in 'interest,' so you are receiving an effective return of 10%.
Interest Rate Supply And Demand
So, the price you are ready to pay for a bond is really equivalent to the principal you are lending out today to receive repayment in the future. Again, the difference between the price you pay today (B) and the amount the bond promises to pay you in the future (A) is equivalent to the interest rate that the bond is effectively going to give you. Or where P is the price you will pay for this bond today, in order to receive an amount of a in t years time, based on an interest rate of r. You can now see algebraically what we demonstrated in words -- the inverse relationship between the bond price and the interest rate.
Let us say you bought a bond which promised to pay $275 in a years time. You bought this bond for $250, which means that implicitly, you would have earned a 10% interest.
Now, let us say that by the time you go to sell this bond, interest rates rose to 20%. This means that no one will want to buy your bond for $250, since this bond only promises to give them $275 or a 10% return at the end of a year. Why will they pay $250 to get $275 at the end of a year, when they can lend out their $250 to someone else and get $300, or a 20% return, at the end of a year?
So, you will not find anyone who is ready to buy your bond for $250. If however, you sell your bond for around $229, then people will be ready to buy it from you. This is because at $229, if they get back $275 at the end of a year, this is almost equivalent to a 20% return.
Thus, if the interest rates go up, the value of your bond will fall from $250 to $229, and you have just made a loss of $21.
Can you see that if the interest rates went down the value of your bond would go up, and you could sell it for more than the $250 you purchased it for? What is the amount you could sell this bond (which promises to pay $275 at the end of the year) for, if interest rates dropped to 5%?
You can try this with a spreadsheet. For example you will find that a bond which pays $1,000 in one year's time would be worth: 3.6 Money Market Equilibrium
What's equilibrium? A situation in which there is no further pressure for change.
Describing equilibrium in the money market will be a matter of describing what the pressures are that will push the interest rate to change. Equilibrium will occur whenever the interest rate stops changing. That will be whenever money supply equals money demand.
Caution: Do not confuse this with a typical micro market equilibrium story of how the price of chicken reaches equilibrium. That's a flow equilibrium : a flow of chicken produced and a flow consumed. This is a stock equilibrium: there is a certain amount of money circulating in the economy, and a certain amount that people wish to hold.
Remember how we discussed equilibrium earlier (in section 1.3). Equilibrium will be a situation in which all the behavioral conditions are satisfied -- when everyone's desired holdings of money equal all the money actually held. An equilibration process will tell us how the money market actually moves to a situation where everybody manages to meet their desired behavior (given from the behavioral functions). The supply of money is the total stock of money available for use in transactions, and held by the private sector. The demand for money balances is the total stock of money that the private sector wishes to hold. Note that when we change the supply of money, as we did in the last chapter, we are changing the amount in deposit accounts. At any instant in time, all the money has to be somewhere: every dollar of the money supply must be held by someone.
Equilibration Stories:
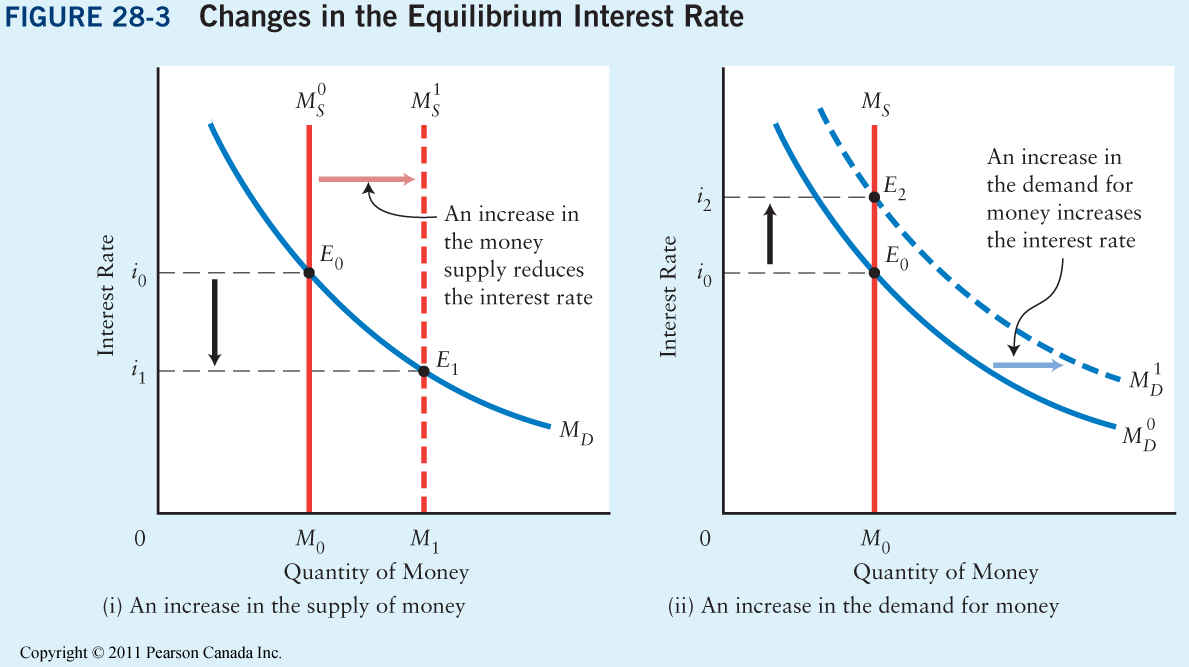
1. Let us suppose that we start with a supply of money that does equal the demand for money, at an interest rate of five percent.
Now we increase the supply of money. That means people now hold more money, relative to bonds, than they used to and want to.
In an effort to readjust their portfolios, they will seek to turn some of this money into bonds -- they'll buy bonds.
This additional demand for bonds will drive up the price of bonds. As you know from the previous section, a higher price of bonds is the same thing as a lower interest rate.
Money Supply And Interest Rates Graph
As the interest rate falls, money demand will rise. Once it rises to equal the new money supply, there will be no further difference between the amount of money people hold and the amount they wish to hold, and the story will end.
This is why (and how) an increase in the money supply lowers the interest rate.
Money Supply And Real Interest Rates Chart
2. Let us suppose that we start with a supply of money that does equal the demand for money, at an interest rate of five percent.
Now we decrease the supply of money. That means people now hold less money, relative to bonds, than they used to and want to.
In an effort to readjust their portfolios, they will seek to turn some of their bonds into money -- they'll sell bonds.
These additional sales of bonds will drive down the price of bonds. As you know from the previous section, a lower price of bonds is the same thing as a higher interest rate.
As the interest rate rises, money demand will fall. Once it falls to equal the new money supply, there will be no further difference between the amount of money people hold and the amount they wish to hold, and the story will end.
This is why (and how) a decrease in the money supply raises the interest rate.
We have a notion of how the interest rate affects demand to hold money which is shown in the downward-sloping money demand curve.
To this, we can add the supply of money. Since it's not affected by the interest rate, it's a vertical line. The Fed fixes the supply of money, as described in the previous chapter. This is the total stock of money in circulation -- the money supply at any given time.
One More Caution: An excess supply of money balances, as occurred when we initially increased the money supply, means that there are more money balances in the economy than individuals desire to hold with them. What are money balances? Money balances are the amount of cash and checking account deposits with the private sector available for use in exchange. In effect, an excess supply of money balances implies that at the going interest rate, there is more cash and a higher level of checking account deposits than individuals desire to hold. This is not the same thing as an 'excess supply of apples,' since an excess supply of apples means that there is a group of people trying to sell apples but unable to do so. An excess supply of money literally means that folks have more cash in their pockets and a larger level of checking accounts than the desire to hold with them.
3.7 Federal Reserve Policy and the Interest Rate
In effect, the Fed can set the interest rate by changing the supply of money. It changes the supply of money by using any of its three instruments -- open market operations, discount rate changes, or required reserve ration changes -- which work on the banking system to increase or decrease the stock of money that is circulating through the economy. The changing supply of money, in turn, changes the interest rate through the process described above.
Caution:The Fed can not 'set' the market interest rate by decree. It can not just tell the money market what the interest rate should be. Do not confuse the discount rate, which the Fed can set, with the interest rate in general. The discount rate is a special rate that banks need to pay if they borrow reserves. It is entirely possible that the discount rate will be substantially higher than the market interest rate. It can also be lower, though in that case the Fed would probably raise it so that banks would not be tempted to use Fed borrowing as a cheap source of funds.
3.8 Other Changes that May Affect the Interest Rate
When we introduced money demand, and in particular transactions demand, the most important part of money demand, we noted that the amount of money that people wished to hold for transactions purposes would be affected by three things:
- a. the interest rate, which represented the opportunity cost of holding money
b. the price level, which would affect how much money was needed for transactions
c. income, because as income rises you buy more, and as it falls you buy less
So if either Y or P (the price level) rise, money demand will increase. Graphically, we represent this as a rightward shift of the money demand curve.
If either Y or P (the price level) fall, money demand will fall. Graphically, we represent this as a leftward shift of the money demand curve.
©1998 S. Charusheela and Colin Danby.